Porosity
Introduction
Porosity is important characteristics of porous body. It is a ratio of the pores volume to the total volume of the porous body.
Method description
It is possible to characterize this parameter by measuring high frequency electric conductivity of the porous body when it is saturated with conducting liquid. Application of high frequency (MHz scale) electric field allows monitoring the full porous space filled with a liquid. Furthermore, it includes dead ends probes, which is impossible for traditional DC and low frequency methods. In addition, it eliminates electrodes polarization, which permits very simple probe design – flat face touching probe as shown on the following photo.

Experimental raw data output of the measurement is so-called “formation factor”. It is a ratio of the porous material conductivity to the conductivity of the liquid that wets this material. There is a well-known and well-verified theory that predicts formation factor. Maxwell and Wagner developed this theory more than hundred years ago. It yields the surprisingly simple expression for the formation factor:
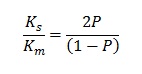
where P is the porosity.
Experimental data
Figure below shows the measured “formation factor” (conductivity ratios Ks/Km) as a function of the total porosity for a series of materials.
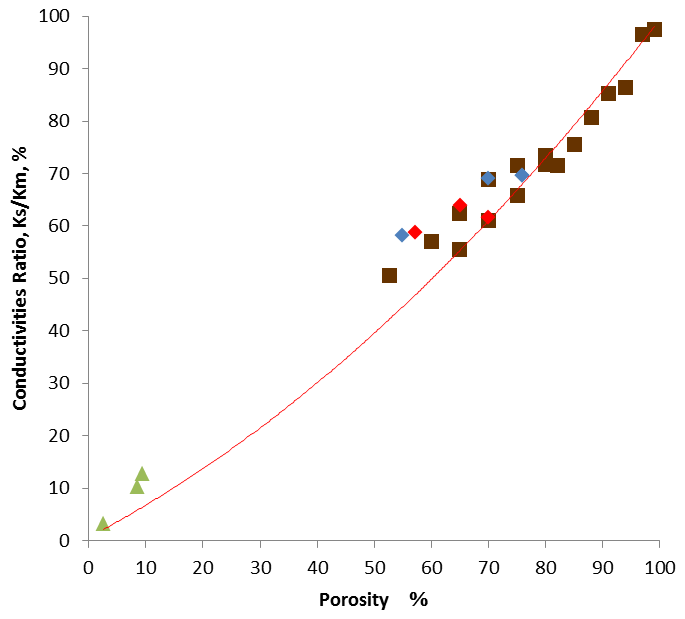
Green triangles correspond to porous sandstones, blue diamonds correspond to CPG porous silica, red diamonds correspond to porous alumina, brown squares correspond to aluminum hydroxide dispersions at different volume fractions prepared with equilibrium dilution. The solid line represents the prediction of Maxwell-Wagner theory.
We would like to stress that this method is applicable for characterizing not only porous monolith, but also porous particles. In the case of particles (either in dispersion or in deposit) it yields the total porosity of the sample, which consists of inter-particle porosity and porosity inside of the particles that includes contribution of all wetted pores even with dead ends. In order to extract porosity of the porous particles one would have to assume inter-particle porosity. We have found that 40% value for this parameter agrees well with independent data.
This method is available with Models DT-330 together with zeta potential measurement. It is available also as an Option OP0014 for Model DT-1202.
Publications
Publications below contain results of this method verification with variety of different porous materials, monoliths and porous particles.
- 1. Dukhin, A.S., Swasey, S. and Thommes, M. “A method for pore size and porosity analysis of porous materials using electroacoustics and high frequency conductivity”, Colloids and Surfaces, (2013).
- 2. Dukhin, A.S., Goetz, P.J. and Thommess, M. “Method for determining Porosity, Pore size And Zeta Potential of Porous Bodies”, patent USA 8,281,662 B2, (2012).
- 3. Dukhin, A.S. and Goetz, J.P. “Characterization of Liquids, Nano- and Microparticulates, and Porous Bodies using Ultrasound”, Edition 3, Elsevier, 571 pages, 765 references, (2017).
