ZETA POTENTIAL and DEBYE LENGTH – A Short Tutorial
Definition of zeta potential
Zeta potential is a parameter characterizing electrochemical equilibrium at interfaces. It depends on the properties of both the surface and the surrounding liquid. It plays an important role in the theory of aggregative stability, also known as DLVO theory[1][2]. Accordingly, electrostatic repulsion between particles is related to the value of zeta potential. The higher the zeta potential, the stronger the repulsion, and therefore the more stable the system becomes. For instance, the high zeta potential of fat droplets in milk prevents them from coalescing. Adding acid to milk, for example, reduces the zeta potential and causes the milk fat droplets to coalesce. Consequently, the formation of cheese is the final result.
Double layer (DL)
Zeta potential is a property of an electric structure that is usually built up at interfaces, in a region referred to as the “double layer” (DL). Simply explained, the double layer is a structure that forms on the surface of an object when it is in a liquid. It consists of two layers: a surface charge layer of ions chemically absorbed onto the surface, and a second layer of oppositely charged ions.
According to Fundamentals of Colloid and Interface Science by J. Lyklema[3]: “…the reason for the formation of a “relaxed” (“equilibrium”) double layer is the non-electric affinity of charge-determining ions for a surface…”. This process leads to the build-up of an electric surface charge. This surface charge creates an electrostatic field that then affects the ions in the bulk of the liquid. This electrostatic field, in combination with the thermal motion of the ions, creates a counter-charge, and thus screens the electric surface charge. The net electric charge in this screening diffuse layer is equal in magnitude to the net surface charge but has the opposite polarity. Consequently, the complete structure is electrically neutral.
Bio-specific non-equilibrium DL.
This definition of the double layer is applicable to equilibrium interfaces and micro-objects. Non-equilibrium micro-objects, like living biological cells, possess additional mechanisms of charge separation at their interfaces. In particular, living biological cells contain ion pumps in their membranes. Consequently, these pumps cause separation of electric charges over the membrane, which leads to additional component of the cell double layer. There are several papers studying such “bio-specific mechanism of double layer formation” theoretically and experimentally [10].
DL structure and Debye length
Figure below shows the distribution of the potential for a positively charged surface.
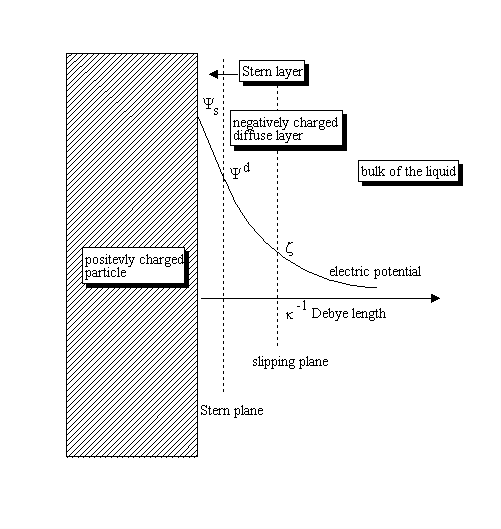 Electric potential decays almost exponentially, which allows introduction of the “Debye length“ as an estimate of the DL thickness. Therefore, electric potential drops by approximately 2.7 times at the distance from the surface that equals to the Debye length. Debye length depends mostly on the “ionic strength” of the liquid and is approximately 1 nm at an ionic strength of 0.1 M. It increases as a reciprocal of the square root of ionic strength (electrolyte concentration) becoming 10 nm at an ionic strength of 0.001 M. Definition of slipping planeThere is another characteristic distance within DL: the location of a slipping plane associated with tangential motion of the liquid relative to the surface. The liquid inside of the slipping plane remains attached to the surface. The liquid beyond the slipping plane moves with the surrounding liquid. The electric potential at the slipping plane is what is referred to as “Zeta potential.” It is measured in millivolts (mV), with a maximum absolute value of 100 mV in aqueous solutions, according to International Standard ISO 13099, Parts 1: 2012 “Colloidal systems – Methods for Zeta potential determination”. DL theoriesCalculation of Zeta Potential from the parameters measured by zeta potential analyzers requires appropriate theory. There are two important asymptotic cases when analytical theories exist. Firstly, the most known case is that of a “thin DL”. It corresponds to the particulates with DLs that are much thinner than their particle radius. The vast majority of aqueous dispersions satisfy this condition, unless for very small particles and low ionic strength media. Calculation of Zeta Potential can be performed using the “Smoluchowski theory” when surface conductivity is negligible. The opposite asymptotic case is that of a “thick DL,” which corresponds to systems where the DL is much larger than the particle radius. The vast majority of dispersions in hydrocarbon media, which inherently have very low ionic strength, satisfy this condition. These two asymptotic cases allow one to picture, at least approximately, the DL structure around spherical particles. DLs overlapThere is one more important factor affecting DL structure: the so-called “overlap of DLs”. Increasing volume fraction and/or reduction of the particle size brings particles surfaces close and eventually diffuse layers would overlap. Therefore, the overlap of Double Layers might become important for nanoparticles, macro-molecules, proteins, etc[6]. It is definitely important in non-polar liquids, when double layers extend quite far and are therefore more likely to overlap. The Double Layers overlap is also important when materials are porous [7][8]. There are more details can be found in the JUPAC report on Electrokinetic phenomena[9], as well as on Wikipedia[11]. An overview of the different methods of Zeta Potential measurement can be found in our book[12]. INTERNATIONAL STANDARDS.REFERENCES.
|
